NEWS: ON December 17, 2023
- Eos3.7.4 was released on December 17, 2023. See the supplements for 3.7.4.
- We started to use file extension wl instead of m (except for init.m).
- The installation of Eos was simplified. The file init.m is automatically generated.
- All the Orikoto programs published on the pages are tested by Eos3.7.4.
News: On October 2, 2023
- Eos3.7.3 was release on October 2. The installation method is the same as that of Eos3.7.2.1. See Supplement for Eos3.7.2.1 for details. The new release has improved functionality of traditional fold.
E-Origami System Eos
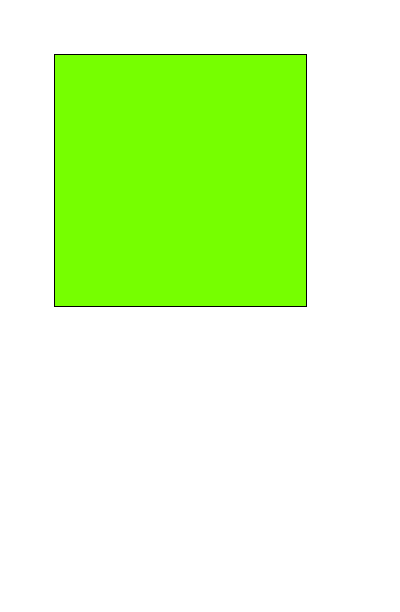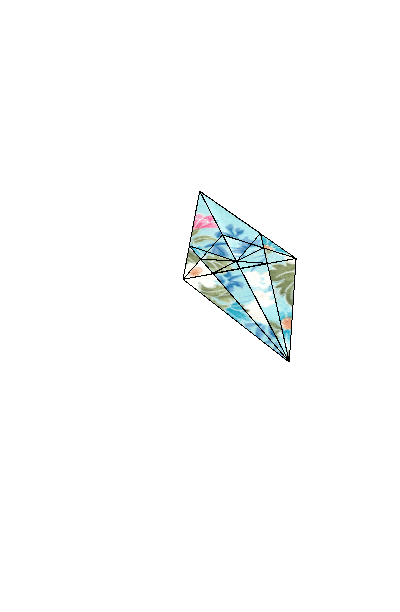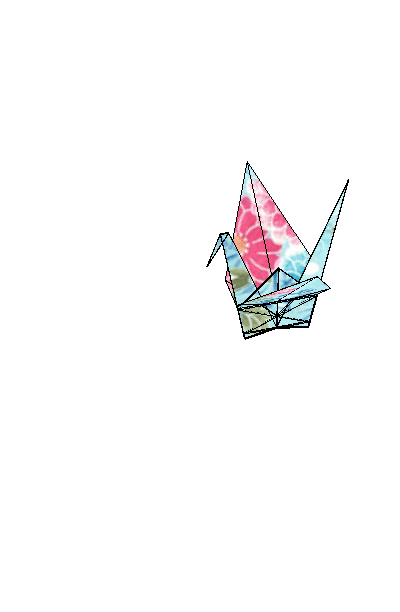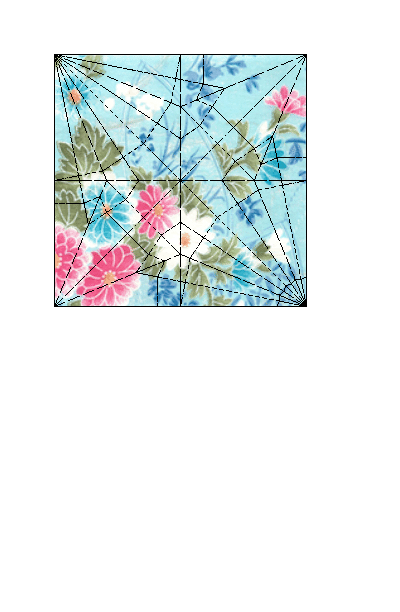
Origami, the art of paper folding, has attracted people’s minds for its beauty and utility in everyday life. Origami has also been the scope of mathematical studies. The folding operations are simple, and easily realized by hand, but powerful enough to solve cubic equations. Origami is regarded as a powerful geometrical tool that allows constructions that classical Euclidean tools could not make.
The computational origami system Eos is designed to study the mathematical aspects of paper folds. It includes capabilities of modeling fold operations by algebraic and symbolic methods, computer simulation of origami construction, and proof of the correctness of the constructed origami. Eos is developed by members of Symbolic Computation Research Group (SCORE), department of computer science of the University of Tsukuba.
- Eos3.32 was released on March 23, 2021. It runs on Mathematica 12.2.
- Eos3.4 was released on May 6, 2021. It runs on Mathematica 12.2.
- Eos3.5 was released on January 20, 2022. It runs on Mathematica 13.
- Eos3.6 was released on July 19, 2022. It runs on Mathematica 13.
- Eos3.7 was released on December 16, 2022. It runs on Mathematica 13.1.
- Eos3.7.1 which was released on March 17, 2023.
- Eos.3.7.2 was released on June 16, 2023:
- Eos.3.7.3 was released on October 2, 2023:
- Eos.3.7.4 was released on December 17, 2023:
Links
